
When the coin is submerged in water as shown in Figure 11.23, its apparent mass is 7.800 g. The mass of an ancient Greek coin is determined in air to be 8.630 g. That isĬalculating Density: Is the Coin Authentic? Alternatively, on balances that measure mass, the object suffers an apparent mass loss equal to the mass of fluid displaced. The object suffers an apparent weight loss equal to the weight of the fluid displaced. This, in turn, means that the object appears to weigh less when submerged we call this measurement the object’s apparent weight. All of these calculations are based on Archimedes’ principle.Īrchimedes’ principle states that the buoyant force on the object equals the weight of the fluid displaced. This same technique can also be used to determine the density of the fluid if the density of the coin is known. The density of the coin, an indication of its authenticity, can be calculated if the fluid density is known. These two measurements are used to calculate the density of the coin.Īn object, here a coin, is weighed in air and then weighed again while submerged in a liquid. (b) The apparent weight of the coin is determined while it is completely submerged in a fluid of known density. The fraction submerged is the ratio of the volume submerged to the volume of the object, orįigure 11.23 (a) A coin is weighed in air. We can derive a quantitative expression for the fraction submerged by considering density. In Figure 11.20, for example, the unloaded ship has a lower density and less of it is submerged compared with the same ship loaded. The extent to which a floating object is submerged depends on how the object’s density is related to that of the fluid. Likewise, an object denser than the fluid will sink. The buoyant force, which equals the weight of the fluid displaced, is thus greater than the weight of the object. This is because the fluid, having a higher density, contains more mass and hence more weight in the same volume. If its average density is less than that of the surrounding fluid, it will float. The average density of an object is what ultimately determines whether it floats. Density and Archimedes’ Principleĭensity plays a crucial role in Archimedes’ principle. (a) What is the mass of this amount of foil? (b) If the foil is folded to give it four sides, and paper clips or washers are added to this “boat,” what shape of the boat would allow it to hold the most “cargo” when placed in water? Test your prediction. Use a piece of foil that measures 10 cm by 15 cm. In equation form, Archimedes’ principle isĪ piece of household aluminum foil is 0.016 mm thick.

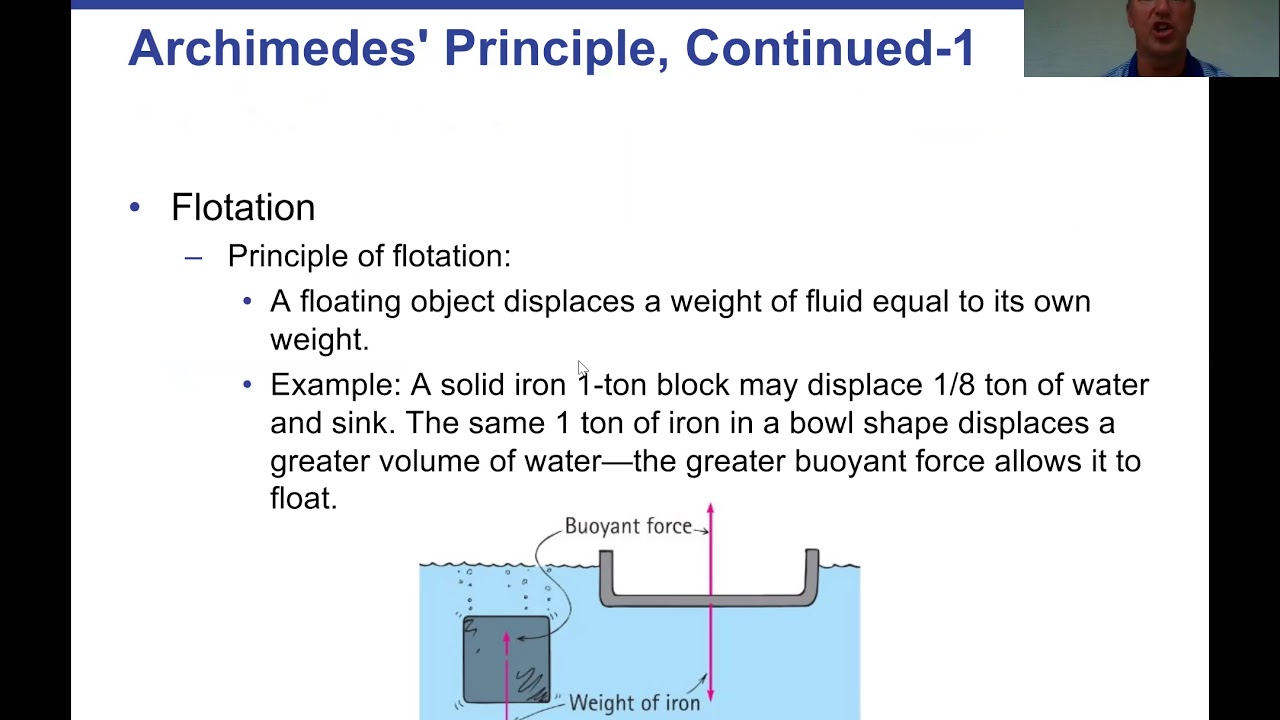
Stated in words, Archimedes’ principle is as follows: The buoyant force on an object equals the weight of the fluid it displaces. 287–212 B.C.) that he stated this principle long before concepts of force were well established. It is a tribute to the genius of the Greek mathematician and inventor Archimedes (ca. This weight is supported by the surrounding fluid, and so the buoyant force must equal w fl w fl, the weight of the fluid displaced by the object. The space it occupied is filled by fluid having a weight w fl w fl. That is, F B = w fl F B = w fl,a statement of Archimedes’ principle.

Since this weight is supported by surrounding fluid, the buoyant force must equal the weight of the fluid displaced. (b) If the object is removed, it is replaced by fluid having weight w fl w fl. If F B F B is less than the weight of the object, the object will sink. If F B F B is greater than the weight of the object, the object will rise. The buoyant force is always present whether the object floats, sinks, or is suspended in a fluid.įigure 11.19 (a) An object submerged in a fluid experiences a buoyant force F B F B. If the buoyant force equals the object’s weight, the object will remain suspended at that depth. If the buoyant force is less than the object’s weight, the object will sink. (See Figure 11.18.) If the buoyant force is greater than the object’s weight, the object will rise to the surface and float. There is a net upward, or buoyant force on any object in any fluid. This means that the upward force on the bottom of an object in a fluid is greater than the downward force on the top of the object. (credit: Crystl)Īnswers to all these questions, and many others, are based on the fact that pressure increases with depth in a fluid. (credit: Allied Navy) (c) Helium-filled balloons tug upward on their strings, demonstrating air’s buoyant effect. (b) Submarines have adjustable density (ballast tanks) so that they may float or sink as desired. Figure 11.17 (a) Even objects that sink, like this anchor, are partly supported by water when submerged.


 0 kommentar(er)
0 kommentar(er)
